|
The model atmosphere are described by a two-dimensional version of the
anelastic system of Ogura and Phillips (1962).
The effect of planetary rotation is not considered.
(A.1), (A.2) are the horizontal and
vertical component of equation of motion, respectively.
(A.3) is the continuity equation
and (A.4) is the
thermodynamic equation.
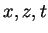 are horizontal, vertical and time
coordinate, respectively. are horizontal, vertical and time
coordinate, respectively.
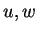 are horizontal and vertical wind velocity, and are horizontal and vertical wind velocity, and
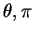 are potential temperature and
nondimensional pressure function deviation from those of basic
state, respectively. are potential temperature and
nondimensional pressure function deviation from those of basic
state, respectively.
 are density, potential temperature and
temperature in basic state. are density, potential temperature and
temperature in basic state.
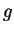 is gravitational acceleration whose value
is equal to 3.72 msec-2. is gravitational acceleration whose value
is equal to 3.72 msec-2.
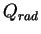 is radiative heating (cooling) rate per
unit mass, which is described in appendix A.d in detail. is radiative heating (cooling) rate per
unit mass, which is described in appendix A.d in detail.
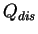 is heating rate per unit mass owing to
dissipation of turbulent kinetic energy, which is given by turbulent parameterization. is heating rate per unit mass owing to
dissipation of turbulent kinetic energy, which is given by turbulent parameterization.
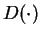 in equation (A.1) 〜 (A.4)
represents the turbulent diffusion owing to subgrid scale turbulent
mixing as follows. in equation (A.1) 〜 (A.4)
represents the turbulent diffusion owing to subgrid scale turbulent
mixing as follows.
| |
|
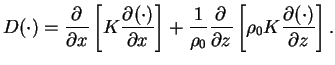 |
(A.5) |
K is turbulent diffusion coefficient which
is calculated by (A.9) and (A.10).
The nondimensional pressure function and potential temperature are
defined as follows.
where 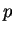 and and 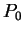 are pressure
and that in basic state, are pressure
and that in basic state, 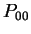 is reference
pressure (= 7 hPa), is reference
pressure (= 7 hPa), 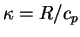 , , 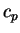 is specific
heat of constant pressure per unit mass and is specific
heat of constant pressure per unit mass and 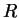 is
atmospheric gas constant per unit mass.
The values of is
atmospheric gas constant per unit mass.
The values of 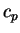 and and 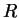 are set to be
those of CO2 (734.9
Jkg-1K-1,
189.0
Jkg-1K-1).
The basic state atmospheric structure is calculated by using
the hydrostatic equation and euation of state for ideal gas as follows. are set to be
those of CO2 (734.9
Jkg-1K-1,
189.0
Jkg-1K-1).
The basic state atmospheric structure is calculated by using
the hydrostatic equation and euation of state for ideal gas as follows.
The perturbation of nondimensional pressure function is diagnosed
by using the following equation which is derived
from (A.1) to (A.3)
|